The displacement
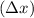 of the compression spring when a 60-kilogram person stands on it, with a spring constant of 2500 N/m, is approximately -0.2352 meters. The negative sign indicates the direction of the displacement. Therefore, the correct answer is D.
of the compression spring when a 60-kilogram person stands on it, with a spring constant of 2500 N/m, is approximately -0.2352 meters. The negative sign indicates the direction of the displacement. Therefore, the correct answer is D.
To find the displacement
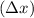 of the spring, you can use Hooke's Law:
of the spring, you can use Hooke's Law:
![\[ F = -k \cdot \Delta x \]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/7reu1pznpc4jdk3dqlcgn3y1l57j1ouf57.png)
Where:
- F is the force applied (weight in this case),
- k is the spring constant, and
-
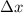 is the displacement of the spring.
is the displacement of the spring.
Rearrange the formula to solve for
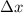 :
:
![\[ \Delta x = -(F)/(k) \]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/9zeerb0g0nuii4xws6kdaldqq5taanvofo.png)
Substitute the given values:
![\[ \Delta x = -(mg)/(k) \]\[ \Delta x = -\frac{(60 \, \text{kg})(9.8 \, \text{m/s}^2)}{2500 \, \text{N/m}} \]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/plggipctmcahh2tu4zyb2vva0q9y2vxa1r.png)
Calculate the result:
![\[ \Delta x \approx -0.2352 \, \text{m} \]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/sj2ovthc86v1ws0kc7vpl395kqpj2xnq8l.png)
The negative sign indicates that the displacement is in the opposite direction to the applied force. Therefore, the correct answer is:
D)
