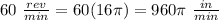Answer:
Part a)
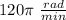
Part b)
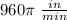
Explanation:
we have
60 rev/min
Part a) Find the angular speed in radians per minute
we know that
One revolution represent 2π radians (complete circle)
so
To convert rev to rad, multiply by 2π
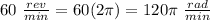
Part b) Find the linear speed in inches per minute
we know that
The circumference of a circle is equal to
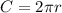
we have
 ----> given problem
----> given problem
substitute
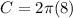
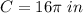
Remember that
One revolution subtends a length equal to the circumference of the circle
so
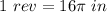
To convert rev to in, multiply by 16π