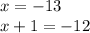Answer:
The integers are -13 and -12
Explanation:
Let
x ----> the first consecutive negative integer
x+1 ----> the second consecutive negative integer
we know that

Apply the distributive property
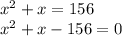
The formula to solve a quadratic equation of the form

is equal to
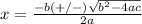
in this problem we have
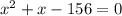
so
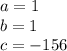
substitute in the formula

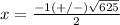
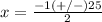
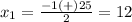 ----> the solution cannot be a positive number
----> the solution cannot be a positive number
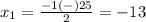
therefore