Question

Please can someone solve this physics question with a good explenation.

Answer 1

The coefficient of dynamic friction is 0.025.

Step-by-step explanation:

Given:

Initial speed after the push is 'v' as seen in the graph.

Final speed of the stone is 0 m/s as it comes to rest.

Total distance traveled is,
`D=29.8\ m`

Total time taken is,
`t_(total)=17.5\ s`

Time interval for deceleration is 3.5 to 17.5 s which is for 14 s.

Now, average speed of the stone is given as:

`v_(avg)=(D)/(t_(total))=(29.8)/(17.5)=1.703\ m/s`

Now, we know that, average speed can also be expressed as:

`v_(avg)=(v_i+v_f)/(2)\\1.703=(v+0)/(2)\\v=2* 1.703=3.41\ m/s`

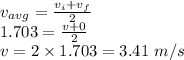
Now, from the graph, the vertical height of the triangles is,
`v=3.41\ m/s`

The deceleration is given as the slope of the line from time 3.5 s to 17.5 s.

Therefore, deceleration is:

`a=\frac{\textrm{Vertical height}}{\textrm{Time interval}}\\a=(v-0)/(17.5-3.5)\\a=(3.41)/(14)=0.244\ m/s^2`

Frictional force is the net force acting on the stone. Frictional force is given as:

`f=\mu_dN\\Where, \mu_d\rightarrow \textrm{coefficient of dynamic friction}\\N\rightarrow \textrm{Normal force}\\N=mg\\\therefore f=\mu_dmg`

Now, from Newton's second law, net force is equal to the product of mass and acceleration.

Therefore,

`\mu_dmg=ma\\\mu_d=(a)/(g)`

Plug in 0.244 for 'a' and 9.8 for 'g'. This gives,

`\mu_d=(a)/(g)=(0.244)/(9.8)=0.025`

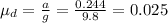
Therefore, the coefficient of dynamic friction is 0.025.