Question

3X squared plus 2X equals -4 number of solutions

Answer 1

The given equation has two solutions i.e
`(-1+√(13) )/(3)` ,
`(-1-√(13) )/(3)`

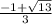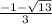
Explanation:

The given expression as :

3 x² + 2 x - 4

For the quadratic equation in the form of a x² + b x + c , the value of x

x =
`\frac{-b\pm \sqrt{b^(2)-4* a* c}}{2* a}`

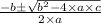
Or, x =
`\frac{-2\pm \sqrt{2^(2)-4* 3* (-4)}}{2* 3}`

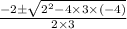
Or, x =
`(-2\pm √(4 + 48))/(6)`

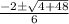
or, x =
`(-2\pm √(52))/(6)`

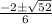
∴ x =
`(-1\pm √(13))/(3)`

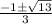
Or, x =
`(-1+√(13) )/(3)` ,
`(-1-√(13) )/(3)`

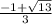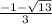
Hence The given equation has two solutions i.e
`(-1+√(13) )/(3)` ,
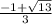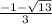`(-1-√(13) )/(3)` Answer