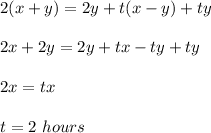Question

A raft and a motorboat simultaneously leave from the same point and move down the river. After 2 hours the motorboat turns around and starts moving towards the raft. How many hours will they move towards each before meeting?

Answer 1

2 hours

Explanation:

Let x mph be the speed of motorboat in still water and y mph be the speed of current.

A raft and a motorboat simultaneously leave from the same point and move down the river, then speeds down the river are:

motorboat = x + y mph

raft = y mph

In two hours they will cover:

motorboat = 2(x+y) miles

raft = 2y miles

After 2 hours the motorboat turns around and starts moving towards the raft (against the current), so its speed is now x - y mph.

Let t be the time they move towards each other. In t hours, they cover

motorboat = t(x - y) miles

raft = ty miles

The distance from the starting point to the turning point is the same as the sum of the distances the raft covers and the motorboat covers after turning around, so

`2(x+y)=2y+t(x-y)+ty \\ \\2x+2y=2y+tx-ty+ty\\ \\2x=tx\\ \\t=2\ hours`