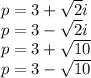Answer:
There are four roots of
 ,
,
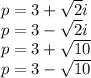
Step-by-step explanation:
Given:

Let
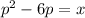
Now solving the equation we get:

Now, solving for two values of
 , we get;
, we get;

Now re-substituting value of
 , we get;
, we get;
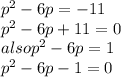
Now we have two quadratic equation we solve for each,
1) We will solve for
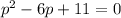
Using quadratic formula to solve it. This gives,

Here
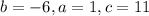
hence,
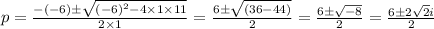
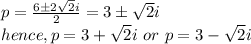
2) We will solve for

Using quadratic formula to solve it. This gives,

here
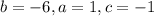
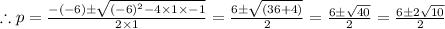
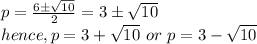
Hence, from 1 and 2, we find there are 2 real roots and 2 imaginary roots.