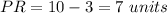Answer:
Part 1)
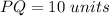
Part 2)
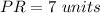
Explanation:
The correct question is
The triangles MNO and PQR are congruent. (MNO≅PQR)
Find the lengths of PQ and PR.
we know that
If two figures are congruent, then its corresponding sides are congruent
In this problem
MN and PQ are corresponding sides
MO and PR are corresponding sides
NO and QR are corresponding sides
therefore
MN≅PQ
MO≅PR
NO≅QR
step 1
Find the value of y
we have
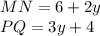
equate the equations
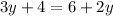
solve for y
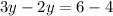
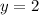
step 2
Find the length of PQ
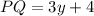
substitute the value of y
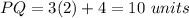
step 3
Find the value of x
we have
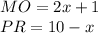
equate the equations
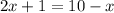
solve for x
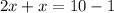
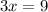

step 4
Find the length of PR
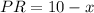
substitute the value of x