Question

A coordinate grid with 2 lines. One line, labeled f(x) passing through (negative 2, 4), (0, 2), and the point (1, 1). The other line is labeled g(x) and passes through (negative 3, negative 3), (0, 0) and the point (1, 1). Which input value produces the same output value for the two functions on the graph? x = −1 x = 0 x = 1 x = 2

Answer 1

x=1

Explanation:

Answer 2

The input value is x=1

Explanation:

we know that

The linear equation in slope intercept form is equal to

`y=mx+b`

where

m is the slope

b is the y-intercept

step 1

we have

f(x) passing through the points

(-2,4),(0,2) and (1,1)

we know that

The formula to calculate the slope between two points is equal to

`m=(y2-y1)/(x2-x1)`

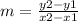
take the points

(0,2) and (1,1)

substitute

`m=(1-2)/(1-0)`

`m=(-1)/(1)`

`m=-1`

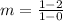
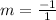
The y-intercept is the point (0,2)

so

`b=2`

substitute

`f(x)=-x+2` ----> equation A

step 2

we have

g(x) passing through the points

(-3,-3),(0,0) and (1,1)

Find the slope

This is a linear direct variation, because the line passes through the origin

so

The equation is

`y=kx`

Find the constant of proportionality k

`k=(y)/(x)`

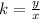
take the point

(1,1)

substitute

`k=(1)/(1)=1`

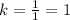
substitute

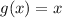`g(x)=x` ----> equation B

The solution of the system of equations A and B is the intersection point both graphs

The intersection point is (1,1) -----> given problem

That means----> For an input value of x=1, the output value in both functions is the same

therefore

The input value is x=1