Question

7. A cube sits in the Cartesian coordinate system. Each side of the cubeis 2m. Electric charge is distributed in the cube with a density functionV=xy2e2z(C=m3). Find the total charge in the cube

Answer 1

Net charge inside the cube is 321.6 C

Step-by-step explanation:

Given charge density function is ρ =
`xy^2 e^(2z)`

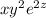
side of cube is 2m

According to the definition of volume charge density total charge is given by

q = total charge =
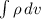`\int {\rho} \, dv` where dv = dxdydz is the volume element

on substututing the respected values we get

q =
`\int\limits^2_0 {x} \, dx \int\limits^2_0 {y^2} \, dy \int\limits^2_0 {e^2^z} \, dz`

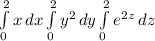
on solving the above integration we get

q =
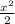`(x^2)/(2)` x
`(y^3)/(3)` x
`(e^2^z)/(2)`

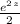
⇒ q = 321.6 C

Therefore net charge inside the cube is 321.6 C