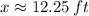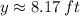Answer:
The dimensions of the pen that minimize the cost of fencing are:
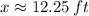
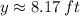
Explanation:
Let
 be the width and
be the width and
 the length of the rectangular pen.
the length of the rectangular pen.
We know that the area of this rectangle is going to be
 .The problem tells us that the area is 100 feet, so we get the constraint equation:
.The problem tells us that the area is 100 feet, so we get the constraint equation:
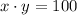
The quantity we want to optimize is going to be the cost to make our fence. If we have chain-link on three sides of the pen, say one side of length
 and both sides of length
and both sides of length
 , the cost for these sides will be
, the cost for these sides will be
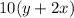
and the remaining side will be fence and hence have cost

Thus we have the objective equation:
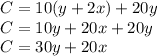
We can solve the constraint equation for one of the variables to get:
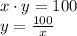
Thus, we get the cost equation in terms of one variable:
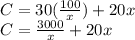
We want to find the dimensions that minimize the cost of the pen, for this reason, we take the derivative of the cost equation and set it equal to zero.
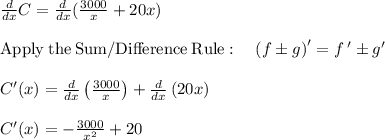
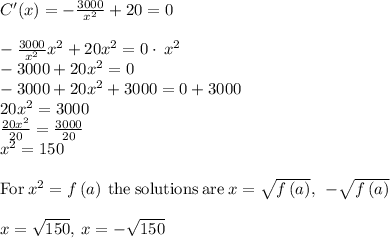
Because length must always be zero or positive we take
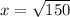 as only value for the width.
as only value for the width.
To check that this is indeed a value of
 that gives us a minimum, we need to take the second derivative of our cost function.
that gives us a minimum, we need to take the second derivative of our cost function.
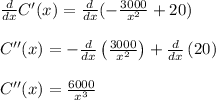
Because
 is greater than zero,
is greater than zero,
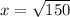 is a minimum.
is a minimum.
Now, we need values of both x and y, thus as
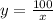 , we get
, we get
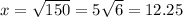
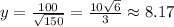
The dimensions of the pen that minimize the cost of fencing are: