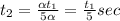Answer:
a)
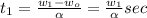
b)
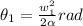
c)
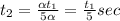
Step-by-step explanation:
1) Basic concepts
Angular displacement is defined as the angle changed by an object. The units are rad/s.
Angular velocity is defined as the rate of change of angular displacement respect to the change of time, given by this formula:
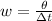
Angular acceleration is the rate of change of the angular velocity respect to the time
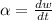
2) Part a
We can define some notation
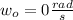 ,represent the initial angular velocity of the wheel
,represent the initial angular velocity of the wheel
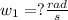 , represent the final angular velocity of the wheel
, represent the final angular velocity of the wheel
 , represent the angular acceleration of the flywheel
, represent the angular acceleration of the flywheel
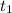 time taken in order to reach the final angular velocity
time taken in order to reach the final angular velocity
So we can apply this formula from kinematics:
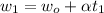
And solving for t1 we got:
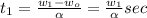
3) Part b
We can use other formula from kinematics in order to find the angular displacement, on this case the following:
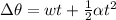
Replacing the values for our case we got:
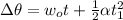
And we can replace
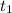 from the result for part a, like this:
from the result for part a, like this:
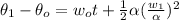
Since
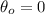 and
and
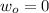 then we have:
then we have:
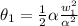
And simplifying:
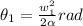
4) Part c
For this case we can assume that the angular acceleration in order to stop applied on the wheel is
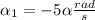
We have an initial angular velocity
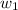 , and since at the end stops we have that
, and since at the end stops we have that
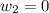
Assuming that
 represent the time in order to stop the wheel, we cna use the following formula
represent the time in order to stop the wheel, we cna use the following formula

Since
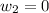 if we solve for
if we solve for
 we got
we got

And from part a) we can see that
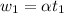 , and replacing into the last equation we got:
, and replacing into the last equation we got: