The sum and difference identity cos(x + y) / sin(x - y) = 1 - cotxcoty / cotx - coty is verified
Solution:
Given expression is:

Let us first solve L.H.S
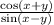 ------ EQN 1
------ EQN 1
We have to use the sum and difference formulas
cos(A + B) = cosAcosB – sinAsinB
sin(A - B) = sinAcosB – cosAsinB
Applying this in eqn 1 we get,

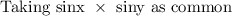
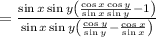
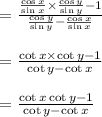
Taking -1 as common from numerator and denominator we get,
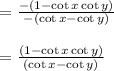
= R.H.S
Thus L.H.S = R.H.S
Thus the given expression has been verified using sum and difference identity