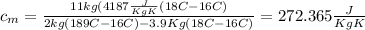Question

Calculate the specific heat of a metal from the following data. A container made of the metal has a mass of 3.9 kg and contains 11 kg of water. A 2.0 kg piece of the metal initially at a temperature of 189°C is dropped into the water. The container and water initially have a temperature of 16.0°C, and the final temperature of the entire system is 18.0°C.

Answer 1

`c_m =272.365(J)/(KgK)`

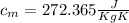
Step-by-step explanation:

1) Notation and data given

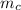`m_c`= mass of the container = 3.9 kg

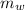`m_w`= mass of the water inside of the container= 11 kg

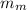`m_m`=mass of the metal= 2 kg

`T_(im)=189\degree C` initital temperature of the metal

`T_(iw)=16\degree C` initital temperature of the water

`T_(ic)=16\degree C` initital temperature of the container

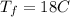`T_(f)=18\degree C` final equilibrium temperature

2) Concepts and formulas

Since the inital temperature for the pice of metal is higher than the temperature for the container and the water inside, the piece of metal will transfer heat, and this transferred heat is the same amount absorbed by the container and the water reaching the equilibrium. So then we have this formula:

`Q_m =Q_w + Q_c`

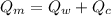
We don't have any change of phase so then we just have the presence of sensible heat, and using this we got that:

`m_p c_m \Delta T_m =m_w c_w \Delta T +m_c c_c \Delta T`

On this case the container is made by the same metal so then
`c_c=c_m`

`m_p c_m \Delta T_m =m_w c_w \Delta T +m_c c_m \Delta T`

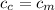
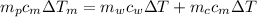
And solving for
`c_m` from the last expression we got:

`m_p c_m \Delta T_m -m_c c_m \Delta T_c =m_w c_w \Delta T`

`c_m [m_p \Delta T_m -m_c \Delta T_c] =m_w c_w \Delta T`

`c_m=(m_w c_w \Delta T)/(m_p \Delta T_m -m_c \Delta T_c)`

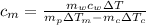
Replacing the values we have:

`c_m=(11kg(4187(J)/(KgK)(18\degree C-16\degree C))/(2kg(189\degree C -16 \degree C) -3.9Kg(18\degree C -16\degree C))= 272.365(J)/(Kg K)`