Question

Delaney would like to make a 10 lb nut mixture that is 60% peanuts and 40% almonds. She has several pounds of peanuts and several pounds of a mixture that is 20% peanuts and 80% almonds. Let p represent the number of pounds of peanuts needed to make the new mixture, and let m represent the number of pounds of the 80% almond-20% peanut mixture.

a) What is the system that models this situation?
b) Which of the following is a solution to the system: 4 lb peanuts and 6 lb mixture; 5 lb peanut and 5 lb mixture; 8 lb peanut and 2 lb mixture? Show your work.

Answer 1

{a}p + 0.2m = 6

0.8m = 4

or 5p + m = 30

m = 5

{b} 5 lb peanuts and 5 lb mixture

Explanation:

m1 → desired mixture with composition : 60% peanuts and 40% almonds and weight : 10 lb

⇒weight of peanuts in m1 = 60% × 5 = 6 lb

and weight of almonds in m1 is 4 lb

m2 → mixture with composition : 20% peanuts and 80% almonds and weight : m pounds

⇒weight of peanuts in m2 = 0.2m

and weight of almonds in m2 = 0.8m

p → weight (in pounds) of peanuts added to m2 to make m1

{a}

add p pounds of peanuts to m2 to make m1

equating weights of peanuts and almonds:

p + 0.2m = 6

0.8m = 4

{b}

equation gives m =
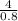`(4)/(0.8)` ⇒ m = 5 lb

Using this in the
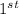`1^(st)` equation:

p + 1 = 6 ⇒ p = 5 lb

Answer 2

a) p + m = 10 and
`(p + 0.2m)/(0.8m) = (60)/(40)`

b) The mixing amount is 5 lb peanut and 5 lb mixture.

Explanation:

Given that p pounds of peanuts and m pounds of the 80% almond and 20% peanut mixture are used to make 10 pounds of 60% peanuts and 40% of almonds mixture.

Now, we can write that p + m = 10 ........ (1)

Now, m pounds of 80% almond and 20% peanut mixture contain 0.2m pounds of peanuts and 0.8m pounds of almonds.

Now, from the condition given it can be written that

`(p + 0.2m)/(0.8m) = (60)/(40)` .......... (2)

⇒
`(p + 0.2m)/(0.8m) = (3)/(2)`

⇒ 2p + 0.4m = 2.4m

⇒ 2p = 2m

⇒ p = m

Now from equation (1) we get p = m = 5 pounds.

a) Therefore, equations (1) and (2) are the system of equations that models the situation.

b) The mixing amount is 5 lb peanut and 5 lb mixture. (Answer)