Answer:
(12,0) and (3, -1) and (0,-4/3)
Explanation:
To find if a point makes an equation true, just substitute its x and y values in in the equation and simplify.
1) Let's test the point (12,0). Substitute 12 for x and 0 for y in the equation:
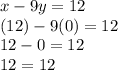
12 does equal 12, so (12,0) makes the equation true.
2) Let's test the point (0, 12). Substitute 0 for x and 12 for y in the equation:
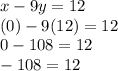
However, -108 does not equal 12, so (0,12) does not make the equation true.
3) Let's test the point (3, -1). Substitute 3 for x and -1 for y in the equation:
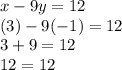
12 does equal 12, so (3, -1) makes the equation true.
4) Let's test the point (0, -4/3). Substitute 0 for x and -4/3 for y in the equation:
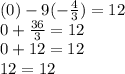
12 does equal 12, so (0, -4/3) makes the equation true.