Question

In a study of red/green color blindness, 550 men and 2400 women are randomly selected and tested. Among the men, 48 have red/green color blindness. Among the women, 5 have red/green color blindness. Test the claim that men have a higher rate of red/green color blindness than women. Also, construct a 98% confidence interval for the difference between color blindness rates of men and women. Does there appear to be a significant difference?

Answer 1

Answer: 98% confidence interval is (0.06,0.11).

Explanation:

Since we have given that

Number of men = 550

Number of women = 2400

Number of men have color blindness = 48

Number of women have color blindness = 5

So,
`p_M=(48)/(550)=0.087\\\\p_F=(5)/(2400)=0.0021`

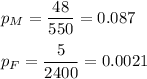
So, at 98% confidence interval, z = 2.326

so, interval would be

`(p_M-P_F)\pm z\sqrt{(p_M(1-p_M))/(n_M)+(p_F(1-p_F))/(n_F)}\\\\=(0.087-0.0021)\pm 2.326\sqrt{(0.087* 0.912)/(550)+(0.0021* 0.9979)/(2400)}\\\\=0.0849\pm 2.326* 0.012\\\\=(0.0849-0.027912, 0.0849+0.027912)\\\\=(0.06,0.11)`

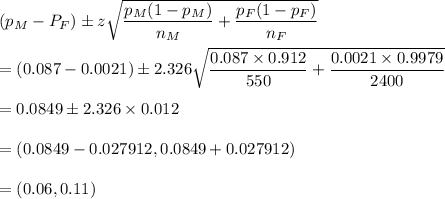
Hence, 98% confidence interval is (0.06,0.11).

No, there does not appears to be a significant difference.