Question

The gateway arc in st.louis was built in 1965. it is the talles monument in the united states. the arch can be modeled with the function y=0.00635x^2+4x where x and y are in feet.

a. how high above the grounf is the tallest point of the arch?
b. how far apart are the legs of the arch at thier bases?
c. what are the domain and range of this function?

Answer 1

A) 630 feet

B) 630 feet

C) Domain: [0,630] and Range: [0,630]

Explanation:

The gateway arc in St. Louis was built in 1965. It is the tallest monument in the united states.

The modeled of arc,
`y=-0.00635x^2+4x`

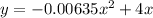
where x and y are in feet

Part A) The given model is parabolic curve. The highest point at vertex of parabolic curve.

The x-coordinate of maximum height,
`x=-(b)/(2a)`

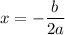
where, a is coefficient of x² and b is coefficient of x

a = -0.00635

b = 4

`x=-(4)/(2* -0.00635)`

`x=314.96`

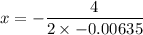
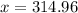
Put x = 314.96 into model to get the value of y

`y_(max)=-0.00635(314.96)^2+4(314.96)`

`y_(max)=629.92\approx 630\text{ feet}`

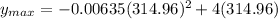
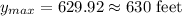
Hence, the tallest point of the arch at 630 feet above ground.

Part B) The distance between the legs of arch is difference of zeros of equation.

`-0.00635x^2+4x=0`

`x=0\text{ and }629.92\approx 630`

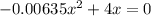
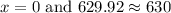
Hence, the legs of the arch 630 feet apart to each other.

Part C)

Domain: It is input value of x where function is defined.

Range: It is output value of function for all defined value of x.

For this model, It is a function of arch. So, y value can't be negative.

Therefore,

Domain: [0,630]

Range: [0,630]