Question

The 2004 landings of the Mars rovers Spirit and Opportunity involved many stages, resulting in each probe having zero vertical velocity about 12 m above the surface of Mars. Determine

(a) the time required for the final free-fall descent of the probes
(b) the vertical velocity at impact. The mass of Mars is 6 . 419 × 10 23 kg , and its radius is 3 . 397 × 10 6 m

Answer 1

2.54334 seconds

9.4364 m/s

Step-by-step explanation:

t = Time taken

u = Initial velocity

v = Final velocity

s = Displacement

a = Acceleration

g = Acceleration due to gravity

G = Gravitational constant = 6.67 × 10⁻¹¹ m³/kgs²

M = Mass of Mars =
`6.419* 10^(23)\ kg`

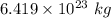
R = Radius of Mars =
`3.397* 10^6\ m`

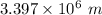
The acceleration due to gravity of a planet is given by

`g=(GM)/(R^2)\\\Rightarrow g=(6.67* 10^(-11)* 6.419* 10^(23))/((3.397* 10^6)^2)\\\Rightarrow g=3.71024\ m/s^2`

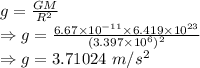
Equation of motion

`s=ut+(1)/(2)at^2\\\Rightarrow 12=0t+(1)/(2)* 3.71024* t^2\\\Rightarrow t=\sqrt{(12* 2)/(3.71024)}\\\Rightarrow t=2.54334\ s`

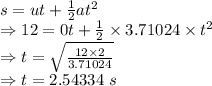
The time taken to fall 12 m in Mars is 2.54334 seconds

`v=u+at\\\Rightarrow v=0+3.71024* 2.54334\\\Rightarrow v=9.4364\ m/s`

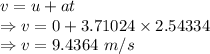
The vertical velocity at impact is 9.4364 m/s