Question

Adidas issued 10-year, 8% bonds with a par value of $200,000. Interest is paid semiannually. The market rate on the issue date was 7.5%. Adidas received $206,948 in cash proceeds. Which of the following statements is true?A. Adidas must pay $200,000 at maturity plus 20 interest payments of $8,000 each.B. Adidas must pay $206,948 at maturity plus 20 interest payments of $8,000 each.C. Adidas must pay $200,000 at maturity plus 20 interest payments of $7,500 each.D. Adidas must pay $200,000 at maturity and no interest payments.E. Adidas must pay $206,948 at maturity and no interest payments.

Answer 1

A. Adidas must pay $200,000 at maturity plus 20 interest payments of $8,000 each.

Step-by-step explanation:

Hi, well it doesn´t matter what the proceeds are, since the bond could be sold at prime (money higher that its face value) or discount (less than its face value), what is really important is the written conditions of this financial instrument, I mean, if a bond has a par value of $200,000, is a 10 year obligation (20 semesters obligation) and pays 8% in coupons (that is typically 8%/2 =4% semi-annual), means that for 20 semesters the issuer of the bond is obligated to pay, $8,000 (this is face value*coupon rate, 200,000*0.04), and when the bond matures (20th semester) the issuer will pay its face value (in our case $200,000) plus the coupon ($8,000).

So, this is what the issuer pays.

From semester 1 to 19 =$8,000

last semester or semester 20 = $208,000

Now, we can check this result by finding the price of this bond, and even though the question does not detail the nature of the discount rate, we have to assume that is 7.5% compounded semi-annually, that is 3.75% effective semi-annually (just take 7.5% and divided by 2). With the following formula, you can find the price of this bond.

`Price=(Coupon((1+YTM)^(n)-1) )/(YTM(1+YTM)^(n) ) +(FaceValue)/((1+YTM)^(n) )`

`Price=(8,000((1+0.0375)^(20)-1) )/(0.0375(1+0.0375)^(20) ) +(200,000)/((1+0.0375)^(20) ) =111,169.63+95,778.47=206,948.10`

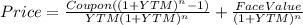
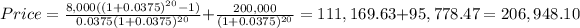
Best of luck.