Answer:
Length of the diagonal for transformed rectangle is 6.40 units.
Explanation:
A rigid transformation is a translation of the image which has same dimensions.
We know that the “length of the rectangle” = 5 units and the width = 4 units.
The units may be any units of length: inches, cm, feet, miles, km etc.
Calculating “the area of the rectangle”:
Area of this rectangle = length x width (of length x breadth) = 5 × 4= 20 square units
Calculating the perimeter of the rectangle
Perimeter of this rectangle = 2 × (length + width) = 2 × (5+4) = 18 units
Calculating the length of the diagonal of the rectangle
The diagonal of this rectangle may be computed using Pythagorean theorem (or Pythagoras Theorem).
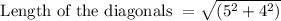
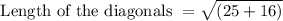
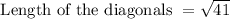
Length of the diagonals = 6.40 units.