Final Answer:
Out of 100 cars on the used car lot:
- 20 cars have manual transmissions,
- 50 cars have air conditioning, and
- 8 cars have both manual transmissions and air conditioning.
Step-by-step explanation:
To determine these figures, we use set theory and the information provided. Let's denote:
- M as the set of cars with manual transmissions,
- A as the set of cars with air conditioning, and
- B as the set of cars with both manual transmissions and air conditioning.
From the information given, we know:
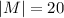 (number of cars with manual transmissions),
(number of cars with manual transmissions),
 (number of cars with air conditioning), and
(number of cars with air conditioning), and
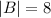 (number of cars with both features).
(number of cars with both features).
We can use the principle of inclusion-exclusion to find the total number of cars with either manual transmissions or air conditioning or both:
![\[|M \cup A| = |M| + |A| - |B| = 20 + 50 - 8 = 62\]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/3j49jbbx6nbhiluvqz6r0w5qaj0hm1yshx.png)
This means that 62 cars out of 100 have either manual transmissions or air conditioning or both.
To find the number of cars with neither manual transmissions nor air conditioning, we subtract this from the total:
![\[|M' \cap A'| = 100 - |M \cup A| = 100 - 62 = 38\]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/i353498j8b94t57emronps3sbq23bo6tt6.png)
So, there are 38 cars on the used car lot without manual transmissions or air conditioning.