Question

Sara sells homemade blueberry jelly (b) and strawberry jam (s) at the farmer's market. It is the end of berry picking season, so

she has enough berries for no more than 40 jars. The blueberry jelly sells for $5 per jar, and the strawberry jam sells for $4 per jar.
Sara's goal is to make at least $120. Which system of inequalities models this situation?​

Answer 1

System of inequalities representing given scenario is as follows

x + y ≤40

5x + 4y ≥ 120

Solution:

Given that Sara sells homemade blueberry jelly (b) and strawberry jam (s) at the farmer's market. She has enough berries for no more than 40 jars.

The blueberry jelly sells for $5 per jar,

And the strawberry jam sells for $4 per jar.

Sara's goal is to make at least $120

Need to write system of inequalities for above scenario.

Let’s assume number of jars of blueberry jelly be represented by variable “x”

And assume number of jars of strawberry jam be represented by variable “y”

Let’s create first inequality

As it is given that Sara has enough berries for no more than 40 jars, so total number of jars cannot be more than 40.

=> Number jars of blueberry jelly + number of jars of strawberry jam ≤40

=> x + y ≤ 40 ------(1)

Now let’s create second inequality

Selling Price of 1 blueberry jelly = $5

So Selling Price of "x" blueberry jelly
`=x * 5=5 x`

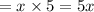
Selling Price of 1 strawberry jam = $4

So Selling Price of "y" strawberry jam =
`\$ 4 * \mathrm{y}=4 y`

So amount generated by selling x blueberry jelly and y strawberry jam = 5x + 4y

And as Sara’s goal is to generate at least $120, so amount generated by selling “x” blueberry jelly and “y” strawberry jam must be greater than $120

=> 5x + 4y ≥ 120 ------ (2)

Hence system of inequalities representing given scenario is as follows :

x + y ≤40

5x + 4y ≥ 120