Question

A solution of 5% acid is mixed with a solution of 6.5% acid to make 200 mL of 6% acid. How many mLs of each of the original solutions are used?

133.33 mL of 6.5% and 66.67 of 5%

Answer 1

66.67 ml of 5% solution and 133.33 ml of 6.5% solution are mixed.

Explanation:

Let 200 ml of 6% solution is formed by mixing x ml of 5% solution and y ml of 6.5% solution.

We can write the equation x + y = 200 ........ (1)

Now, x ml of 5% solution will contain
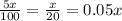`(5x)/(100) = (x)/(20) = 0.05x` ml of acid

Again, y ml of 6.5% solution will contain
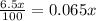`(6.5x)/(100) = 0.065x` ml of acid.

So, mixing them together will give (0.05x + 0.065y) ml of acid in 200 ml of solution.

So, from the condition given we can write

`(0.05x + 0.065y)/(200) = (6)/(100)`

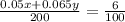
⇒ 0.05x + 0.065y = 12

⇒ x + 1.3 y = 240 ........... (2) {Dividing both sides by 0.05}

Now, solving equations (1) and (2), we get

0.3y = (240 - 200) = 40

⇒ y = 133.33 ml

From equation (1) we get, x = 200 - 133.33 = 66.67 ml

Therefore, 66.67 ml of 5% solution and 133.33 ml of 6.5% solution are mixed. (Answer)