Question

A food company distributes its tomato soup in two cans of different sizes. For the larger can, both the diameter and the height have been increased by 20% .

By what percentage does the volume of the can increase from the smaller can to the larger can? Round your answer to the nearest percent.

Answer 1

73%

Explanation:

If the diameter (d) and height (h) of the small can is increased by 20%, then the diameter (D) of the large can will be 1.2d and the height of the large can (H) will be equal to 1.2h.

Now, the volume of the small can,
`v = \pi ((d)/(2)) ^(2) h = (\pi d^(2) h )/(4)`.

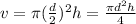Now, the volume of the large can will be
`V = \pi ((D)/(2) )^(2) H = (\pi D^(2) H )/(4) = (\pi (1.2d)^(2) * (1.2h) )/(4) = 1.728 * (\pi d^(2) h )/(4)`

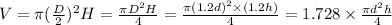
Therefore, the percentage increase in volume from smaller can to larger can will be
`(1.728 * (\pi d^(2) h )/(4) - (\pi d^(2) h )/(4))/((\pi d^(2) h )/(4)) * 100`

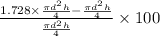
= 72.8%

≈ 73% (Answer)