The equation of line passing through (-8, -2) and (-4, 6) is y = 2x + 14
Solution:
Given that
Line is passing through point (− 8 ,− 2) and ( -4 , 6 )
Equation of line passing through point
 is given by:
is given by:
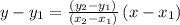 ----- eqn 1
----- eqn 1
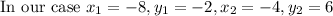
Substituting given value in (1) we get
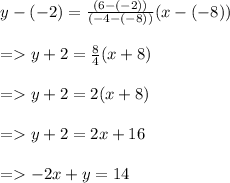
Thus the required equation of line is y = 2x + 14