Equation of a line with an slope of 1/2 that contains (-4, 7) is
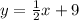
Solution:
We have been given a point and slope of an equation and have been asked to write it in an equation. The two forms of writing a point and slope in equation are point slope form and standard form.
The standard form of a line is in the form Ax + By = C where A is a positive integer, and B, and C are integers. The standard form of a line is just another way of writing the equation of a line.
To write in standard form we will first write it in point slope form and then rearrange it into a standard from.
The formula for point slope form:
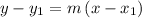
where "m" is the slope of line
Given that "m" =
 and
and
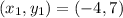

Now, let us convert this equation to standard form
0.5x + 9 = y
0.5x - y = -9
Thus we have found out equation of line in point slope and standard form