Question

Determine whether the set {x2 + 2x − 3, 4x2 + x − 1, 5x2 + 4x − 4} is a basis for P2 by solving the equation a(x2 + 2x − 3) + b(4x2 + x − 1) + c(5x2 + 4x − 4) = 0 for a, b, and c.

Answer 1

The set is a basis for P2

Explanation:

Remember, a set B is a basis for a vector space V if B is a linear independent set and gen(B)=V. Also, remember that the polynomials
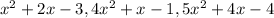`x^2+2x-3, 4x^2+ x-1, 5x^2+4x-4` are linearly independent if the unique scalars
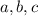`a,b,c` such that

`a(x^2+2x-3)+b(4x^2+ x-1)+c(5x^2+4x-4)=0`

are
`a=b=c=0`

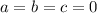
Expanding the above equation we have that

`ax^2+2ax-3a+4bx^2+ bx-b+5cx^2+4cx-4c=0\\(a+4b+5c)x^2+(2a+b+4c)x+(-3a-b-4c)=0`

Then we need values of a, b and c such that

`a+4b+5c=0\\2a+b+4c=0\\-3a-b-4c=0`

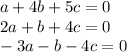
So, we have a homogeneous system with augmented matrix

`A= \left[\begin{array}{ccc}a&4b&5c\\2a&b&4c\\-3a&-b&-4c\end{array}\right]`

Now, we apply row operations for solve the system

1. To the second row of A we subtract the first row twice. And to the third row we add the first row three times. We get the matrix:

`\left[\begin{array}{ccc}a&4b&5c\\0&-7b&-6c\\0&11b&11c\end{array}\right]`

2. We multiply the third row of the previous matrix by 1/11

`\left[\begin{array}{ccc}a&4b&5c\\0&-7b&-6c\\0&b&c\end{array}\right]`

3. To the third row of the previous matrix we add 7 times the second row.
`\left[\begin{array}{ccc}a&4b&5c\\0&-7b&-6c\\0&0&42c\end{array}\right]`

Since the rank of the matrix is 3 then the system has unique solution. But the system is homogeneous, therefore the solution is

`(a,b,c)=(0,0,0)`

This show that the polynomials are linear independent and therefore the set is a basis for P2