Question

a line has a slope of -2/3 for a pair of points on the line. Find the missing coordinate if the points are (-1,y) and (4,7)

Answer 1

The missing co-ordinate is: (-1,
`$ (31)/(3) $`).

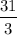Explanation:

Given the slope of a line and a point on it, we determine the equation of the line by slope - one point form.

Slope - one point form: (y - y₁) = m(x - x₁)

Where, (x₁,y₁) is the point passing through the line.

Here the slope is
`$ (-2)/(3) $` and the point (x₁,y₁) = (4,7).

Therefore the equation would be:

y - 7 =
`$ (-2)/(3) $`(x - 4)

⇒ 3y - 21 = -2x + 8

⇒ 2x + 3y - 29 = 0 is the equation of the line.

Now it is given that (-1,y) also passes through the line, this point should satisfy the equation.

⇒ 2(-1) +3y = 29

⇒ 3y = 31 i.e., y =
`$ (31)/(3) $`

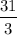
Therefore, the missing co-ordinate is: (-1,
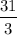`$ (31)/(3) $`).