To solve the problem it is necessary to apply the conservation equations of the moment.
The conservation equation of the moment is defined as,
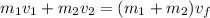
Where,
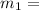 Mass of Bird
Mass of Bird
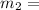 Mass of Insect
Mass of Insect
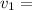 Velocity Bird before swallowing
Velocity Bird before swallowing
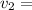 Velocity of insect
Velocity of insect
 final velocity (both)
final velocity (both)
Replacing with our values we have,

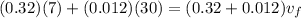
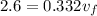
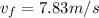
Therefore the bird's speed immediately after swallowing is 7.83m/s