Answer:
The equation of line with given slope that include given points is 3 y + x - 20 = 0
Explanation:
According to Cora , if we know the slope and points on a line then we can write the equation of a line .
Since , The equation of line in slope-intercept form is
y = m x + c
Where m is the slope of line , and if we know the points ( x , y ) which satisfy the line then constant term c can be get and the equation of line can be formed .
So , From the statement said above it is clear that she is correct .
Now , Again
Given as :
Slope of a line is m = -

That include points ( 2 , 6 )
Now from the equation of line as y = m x + c
∴ 6 = -
 ( 2 ) + c
( 2 ) + c
Or, 6 = -
 + c
+ c
So , c = 6 +

or, c =
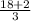
∴ c =
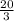
So, The equation of line can be written as
y = -
 x +
x +
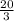
Or, 3 y = - x + 20
I.e 3 y + x - 20 = 0
Hence The equation of line with given slope that include given points is 3 y + x - 20 = 0 Answer