Answer:
Δr=20.45 %
Step-by-step explanation:
Given that
Rake angle α = 15°
coefficient of friction ,μ = 0.15
The friction angle β
tanβ = μ
tanβ = 0.15
β=8.83°
2φ + β - α = 90°
φ=Shear angle
2φ + 8.833° - 15° = 90°
φ = 48.08°
Chip thickness r given as
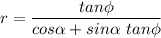
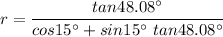
r=0.88
New coefficient of friction ,μ' = 0.3
tanβ' = μ'
tanβ' = 0.3
β'=16.69°
2φ' + β' - α = 90°
φ'=Shear angle
2φ' + 16.69° - 25° = 90°
φ' = 49.15°
Chip thickness r' given as
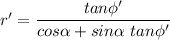
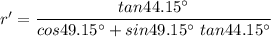
r'=0.70
Percentage change
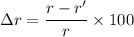
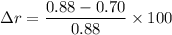
Δr=20.45 %