Question

You are testing the claim that the mean GPA of night students is greater than the mean GPA of day students. You sample 60 night students, and the sample mean GPA is 2.23 with a standard deviation of 0.5 You sample 55 day students, and the sample mean GPA is 2.33 with a standard deviation of 0.62 Calculate the test statistic, rounded to 2 decimal places

Answer 1

Answer: t= -0.95

Explanation:

Given : You are testing the claim that the mean GPA of night students is greater than the mean GPA of day students.

Let
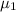`\mu_1` and
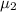`\mu_2` are the mean GPA of night students and mean GPA of day students respectively.

Then, Claim :
`H_a: \mu_1>\mu_2`

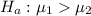
Test statistic for difference between two population mean :

`t=\frac{\overline{x}_1-\overline{x}_2}{\sqrt{(s_1^2)/(n_1)+(s_2^2)/(n_2)}}`

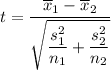
, where

`n_1\ \&\ n_2`= sample standard deviations from population 1 and 2.

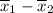`\overline{x}_1-\overline{x}_2` = difference in two sample means.

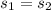`s_1=s_2`= sample standard deviations from population 1 and 2.

As per given , we have

`\overline{x}_1=2.23\ \&\ \overline{x}_2= 2.33`

`n_1=60,\ \ n_2=55`

`s_1=0.5,\ \ s_2=0.62`

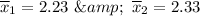
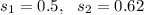
Test statistic :

`t=\frac{2.23-2.33}{\sqrt{((0.5)^2)/(60)+((0.62)^2)/(55)}}`

`t=\frac{-0.1}{\sqrt{(0.25)/(60)+(0.3844)/(55)}}`

`t=(-0.1)/(√(0.0111557575758))`

`t=(-0.1)/(0.1056208198)=-0.946783\approx-0.95`

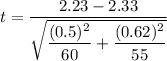
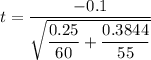
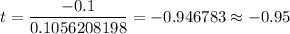
Hence, the test statistic : t= -0.95