Question

Of a random sample of 200 auditors, 105 indicated some measure of agreement with this statement: "Cash flow is an important indicator of profitability." [Assume the sample is large](a)Test at the 10% significance level against a two-sided alternative the null hypothesis that one-half of the members of this population would agree with this statement. (b)Find and interpret the p-value of this test.

Answer 1

a)At the 10% significance level, we fail to reject the null hypothesis.

b) P-value is ≈ 0.48

Explanation:

Let p be the proportion of the auditors agree with the statement "Cash flow is an important indicator of profitability."

Then,

`H_(0)`: p=0.5

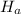`H_(a)`: p≠0.5

Test statistic can be calculated as:

z=
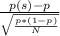`\frac{p(s)-p}{\sqrt{(p*(1-p))/(N) } }` where

• p(s) is the sample proportion of the auditors agree with the statement. (
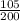  `(105)/(200)`=0.525)

• p is the proportion assumed under null hypothesis. (0.5)

• N is the sample size (200)

Using these numbers we get:

z=
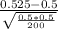`\frac{0.525-0.5}{\sqrt{(0.5*0.5)/(200) } }`≈0,707

and p(z) is ≈ 0.48. Since 0.48>0.10, the result is not significant at 90% level. Therefore we fail to reject the null hypothesis.