Answer:
27.65 sec
Step-by-step explanation:
The Cylinder rotates about it's axis, thus there exists a Centrifugal force on a object which is on the inner surface of the cylinder. This is one of the many ideas that are currently being used in space habitation.
So, Centrifugal force acts like Normal gravity when both have equal magnitude.
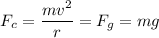
 is the mass of astronaut,
is the mass of astronaut,
 is the Earth's gravity,
is the Earth's gravity,
 is the velocity of astronaut,
is the velocity of astronaut,
 is the Time period of rotation.
is the Time period of rotation.
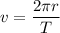
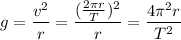
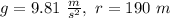
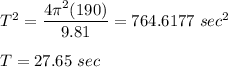
∴ It takes 27.65 sec for rotation of cylinder, if it is to provide normal gravity for astronauts.