Question

One leg of a right triangle is 3 meters shorter than the other leg. If the hypotenuse is 14 meters, find the length of the two legs. Approximate your answers to the nearest tenth of a meter. Answer with the smaller of the legs first.

Answer 1

Answer: Length of one leg =x = 11.3 m

Length of another leg = 11.3-3 = 8.3 m

Explanation:

Let one leg of the right triangle be x , then the other leg be x-3.

Hypotenuse = 14 meters

• Pythagoras theorem of right -triangle says that he square of the length of the hypotenuse is equal to the sum of squares of the lengths of other two sides of the right-angled triangle.

Then for the given situation , we have

`(14)^2=x^2+(x-3)^2\\\\\Rightarrow\ 196=x^2+x^2+3^2-2(3)x\\\\\Rightarrow\ 196=2x^2+9-6x\\\\\Rightarrow 2x^2+9-6x-196=0\\\\\Rightarrow 2x^2-6x-187`

Which is a quadratic equation.

For quadratic equation
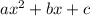`ax^2+bx+c`, the root of equation is
`x=(-b\pm√(b^2-4ac))/(2a)`

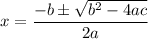
In
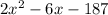`2x^2-6x-187` , a= 2 , b= -6 and c=-187 , then

`x=(-(-6)\pm√((-6)^2-4(2)(-187)))/(2(2))`

`x=(6\pm√(1532))/(4)`

`x\approx(6\pm 39.14)/(4)\\\\ x=(6+39.14)/(4)\ or\ x=(6-39.14)/(4)`

`\\\\ x=11.285\ or\ x=-8.285`

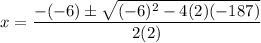
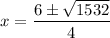
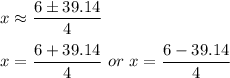
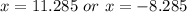
Side cannot be negative , so avoid x=-8.285.

so
`x=11.285\approx11.3`

⇒ Length of one leg =x = 11.3 m

Length of another leg = 11.3-3 = 8.3 m