Answer: 271
Explanation:
The formula we use to find the sample size is given by :-
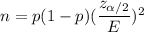
, where
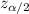 is the two-tailed z-value for significance level of
is the two-tailed z-value for significance level of
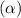
p = prior estimation of the proportion
E = Margin of error.
If prior estimation of the proportion is unknown, then we take p= 0.5 , the formula becomes
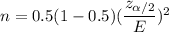
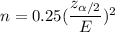
Given : Margin of error : E= 0.05
Confidence level = 90%
Significance level
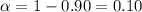
Using z-value table , Two-tailed z-value for significance level of
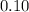
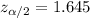
Then, the required sample size would be :
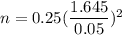
Simplify,
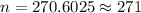
Hence, the required minimum sample size =271