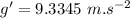Question

The value of g at the height of the space shuttle’s orbit is

Answer 1

`g'=9.3345\ m.s^(-2)`

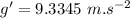
Step-by-step explanation:

The minimum height of a space shuttle from the surface of the earth is 304 kilometers.

So according to this data we calculate the value to g at that height.

We have the formula:

`g'=g(1-(2h)/(R) )`

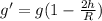
where:

g' = the acceleration due to gravity at the given height 'h' above the earth

R = radius of the earth

g = the acceleration due to gravity at the surface of the earth

Now, using eq. (1)

`g'=9.8(1-(304* 10^3)/(64000* 10^3) )`

`g'=9.3345\ m.s^(-2)`