Answer:
The current is 0.08 ampere.
Explanation:
Given:
The current is 1/2 ampere when the resistance is 400 ohms.
Now, the current I in an electrical conductor varies inversely as the resistance R of the conductor.
So, as per formula
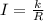
Solving for "k" using the current 1/2 amperes when the resistance is 400 ohms.
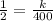
By cross multiplication we get:
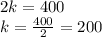
Putting the value of
 for solving the equation:
for solving the equation:
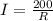
Now, the current when the resistance is 2500 ohms:
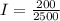

 ampere.
ampere.
Therefore, the current is 0.08 ampere.