Answer: 5y + 4x = - 10
Explanation:
Two lines are said to be perpendicular if the product of their gradients = -1.
If the gradient of the first line is
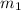 and the gradient of the second line is
and the gradient of the second line is
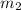 , if the lines are perpendicular, them
, if the lines are perpendicular, them
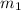 x
x
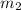 = -1 , that is
= -1 , that is
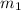 =
=
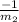
The equation of the line given is 5x - 4y = -3 , we need to write this equation in slope - intercept form in order to find the slope.
The equation in slope -intercept form is given as :
y =mx + c , where m is the slope and c is the y - intercept.
Writing the equation in this form , we have
5x - 4y = + 3
4y = 5x -+3
y = 5x/4 + 3/4
comparing with the equation y = mx + c , then
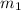 = 5/4
= 5/4
Which means that
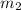 = -4/5 and the line passes through the point ( -5 , 2 ).
= -4/5 and the line passes through the point ( -5 , 2 ).
Using the equation of line in slope - point form to find the equation of the line;
y -
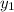 = m ( x -
= m ( x -
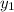 )
)
y - 2 = -4/5 ( x +5)
5(y - 2 ) = -4 ( x + 5 )
5y - 10 = -4x - 20
5y + 4x = - 10