Question

line segments AB has endpoints A(9,3) and B(2,6). Find the coordinates of the point that divides the line segment directed from A to B in the ratio of 1:2.

Answer 1

(x,y) = (20/3 , 4)

Explanation:

(x,y) = (20/3 , 4)

Answer 2

The coordinates of the point that divides the line segment in the ratio of 1:2 is (x,y) = (20/3 , 4)

Explanation:

The end point coordinates of the segment AB is A(9,3) and B(2,6)

Let us assume the point P(x,y) divides the segment AB in the ratio 1: 2

⇒AP : PB = 1: 2

Now, by SECTION FORMULA:

If The point (x,y) divides the line segment with points (x1,y1) and (x2,y2) in the ratio m1: m2, then the coordinates of (x,y) is given as:

`(x,y)  = ({(x_2 m_1 + m_2 x_1)/(m1 + m2) , (y_2 m_1 + m_2 y_1)/(m1 + m2) })`

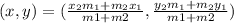
Applying the section formula in the given cindition,

here m1 :m2 = 1 :2

we get,
`(x,y)  = ({(2(1) + 2(9) )/(1 +2) , (6(1)  + 2(3))/(1 + 2) })\\\implies (x,y)  = ((2 + 18)/(3) ,(6 + 6)/(3))\\ \implies (x,y) = ((20)/(3), (12)/(3)  )`

Now, comparing each ordinate separately

`x  =    (20)/(3)       ,y  =  (12)/(3)  = 4`

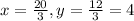
⇒ The coordinates of P(x,y) = (20/3 , 4)

Hence, the coordinates of the point that divides the line segment in the ratio of 1:2 is (x,y) = (20/3 , 4)