Question

An airplane has mass of 5,320 kg. At takeoff, how much lift force is necessary to accelerate the plane upward at a rate of 1.40m/ to the second power?

Answer 1

59637.2 N force is required to lift the plane upward.

Step-by-step explanation:

Mass of the aeroplane (m) = 5320 kg

Acceleration of the plane upward at a rate (a) = 1.40 m/s

"Newton's second law of motion", F - R = ma, where, "force" (F) acting on an object, (R) "resistive force", "mass" (m) of an object and "acceleration" (a).

Force = Resistive force + (mass × acceleration)

Resistive force = mass of an object × acceleration due to gravity. ( we know that acceleration due to gravity is
`\left.9.8 \mathrm{m} / \mathrm{s}^(2)\right)`

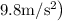
Resistive force = 5320 × 9.81

Resistive force = 52189.2 N

Force = 52189.2 + (5320kg × 1.40 m/s)

Force = 59637.2 N

At takeoff, 59637.2 N force is necessary to accelerate the plane upward at a rate of 1.40 m/s .