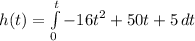Answer with Step-by-step explanation:
Since we have given that
Initial velocity = 50 ft/sec =

Initial height of ball = 5 feet =
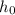
a. What type of function models the height (ℎ, in feet) of the ball after tt seconds?
As we know the function for height h with respect to time 't'.
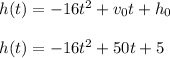
b. Explain what is happening to the height of the ball as it travels over a period of time (in tt seconds).
What function models the height, ℎ (in feet), of the ball over a period of time (in tt seconds)?
if it travels over a period of time then time becomes continuous interval . so it will use integration over a period of time
Our function becomes,