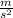Answer:
5.92
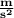
Step-by-step explanation:
The motion of the car can be described as uniform motion in a circle. Hence, speed of the car is assumed constant.
She completes 12 laps in 4 minutes. So each lap takes 20 seconds.
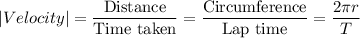
Radius of lap =
 .
.
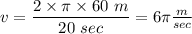
Mass of car =
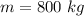
Centripetal acceleration =

∴ Centripetal Acceleration = 5.92