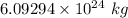Question

A satellite orbits the Earth in an elliptical orbit. At perigee its distance from the center of the Earth is 22500 km and its speed is 4280 m/s. At apogee its distance from the center of the Earth is 24100 km and its speed is 3990 m/s.using this information calculate the mass of the earth. please explain how you arrived to your answer. I have an exam coming up.

Answer 1

`6.09294* 10^(24)\ kg`

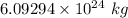
Step-by-step explanation:

K = Kinetic energy

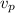`v_p` = Perigee speed = 4280 m/s

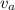`v_a` = Apogee speed = 3990 m/s

`r_p` = Perigee Distance = 22500000 m

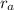`r_a` = Apogee Distance = 24100000 m

G = Gravitational constant = 6.67 × 10⁻¹¹ m³/kgs²

M = Mass of Earth

m = Mass of satellite

In this system the kinetic and potential energies are conserved

`K_p+P_p=K_a+P_a\\\Rightarrow (1)/(2)mv_p^2-(GMm)/(r_p)=(1)/(2)mv_a^2-(GMm)/(r_a)\\\Rightarrow (1)/(2)m(v_p^2-v_a^2)+GMm\left((1)/(r_a)-(1)/(r_p)\right)=0\\\Rightarrow M=(v_a^2-v_p^2)/(2G)* \left((1)/(r_a)-(1)/(r_p)\right)^(-1)\\\Rightarrow M=(3990^2-4280^2)/(2* 6.67* 10^(-11))* \left((1)/(24100000)-(1)/(22500000)\right)^(-1)\\\Rightarrow M=6.09294* 10^(24)\ kg`

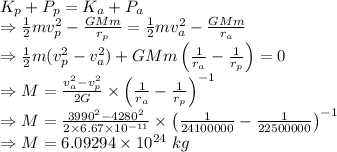
The mass of the Earth is
`6.09294* 10^(24)\ kg`