Question

Suppose a three-seat city council election. For each seat, one progressive and one conservative is running, and voters must choose between one of the two candidates. Further suppose there are more conservative than progressive voters, such that the probability of a conservative candidate winning is 0.6. (a) What is the probability that the conservative party wins all three seats? (b) What is the probability that the conservative party wins exactly two seats?

Answer 1

The probability that conservative party wins all 3 seats is 0.216

The probability that conservative party wins exactly two seats is 0.432

Explanation:

Consider the provided information.

The probability of a conservative candidate winning is p=0.6.

The probability of one progressive candidate will win is: 1-0.6=0.4

Part (a) What is the probability that the conservative party wins all three seats?

According to binomial distribution:
`b(x; n, p) =^nC_xp^x(1- p)^(n-x)`

`P(x=3) =^3C_3(0.6)^3 (0.4)^(3-3)`

`P(x=3) =(0.6)^3`

`P(x=3)=0.216`

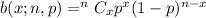
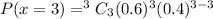
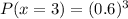
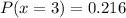
P(conservative party wins all 3 seats) = 0.216

Hence, the probability that conservative party wins all 3 seats is 0.216

Part (a) What is the probability that the conservative party wins exactly two seats?

`P(x=2) =^3C_2(0.6)^2 (0.4)^(3-2)`

`P(x=2) =3(0.36)(0.4)`

`P(x=2) =0.432`

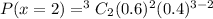
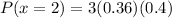
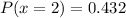
Hence, the probability that conservative party wins exactly two seats is 0.432