Answer: a) The percent of change in perimeter is 100%.
b) The percent of change in area is 300%.
Explanation:
Since we have given that
if the side lengths of rectangle get doubled.
Let the length be 'x'.
Let the breadth be 'y'.
So, Perimeter of rectangle would be

Area of rectangle would be
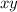
After doubling the lengths,
Length becomes '2x'.
Width becomes '2y'.
So, perimeter becomes
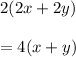
Area becomes
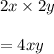
a. Find the percent of change in the perimeter.
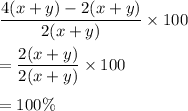
Hence, the percent of change in perimeter is 100%.
b. Find the percent of change in the area.
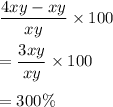
Hence, the percent of change in area is 300%.