Question

given f(x)=3^x-2 and g(x)=f(3x)+4, write the function rule for function g and describe the types of transformations that occur between function f and function g.

Answer 1

`g(x)=3^(3x)+2`

The transformation that occurs from
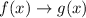`f(x)\rightarrow g(x)` is compression by 3 units horizontally and shift of 4 units upwards.

Explanation:

Given

`f(x)=3^x-2`

`g(x)=f(3x)+4`

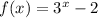
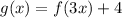
Translation Rules:

`f(x)\rightarrow f(cx)`

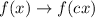
If
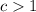`c>1` the function is compressed
`c` units horizontally.

If
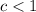`c<1` and
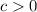`c>0` the function stretches
`c` units horizontally.

`f(x)\rightarrow f(x)+c`

If
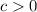`c>0` the function shifts
`c` units to the up.

If
`c<0` the function shifts
`c` units to the down.

Applying the rules to
`f(x)`

Step 1

`f(x)\rightarrow f(3x)`

`f(3x)=3^(3x)-2`

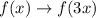
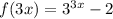
[compressed
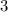`3` units horizontally]

Step 2

`f(3x)\rightarrow f(3x)+4=g(x)`

`f(3x)+4=3^(3x)-2+4`

∴
`g(x)=3^(3x)+2`

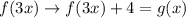
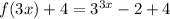
[shifts 4 nits up]

∴ The transformation that occurs from
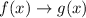`f(x)\rightarrow g(x)` is compression by 3 units horizontally and shift of 4 units upwards.