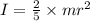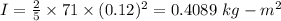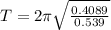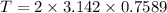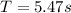Question

A 71 kg solid sphere with a 12 cm radius is suspended by a vertical wire. A torque of 0.41 N·m is required to rotate the sphere through an angle of 0.76 rad and then maintain that orientation. What is the period of the oscillations that result when the sphere is then released?

Answer 1

Answer:5.47 s

Step-by-step explanation:

Given

mass of solid sphere
`m=71 kg`

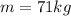
radius of sphere
`r=12 cm`

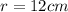
Torque Applied
`T=0.41 N-m`

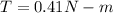
Inclination Produced
`\theta =0.76 rad`

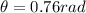
Now we know that time Period of Torsion Pendulum

`T=2\pi \sqrt{(I)/(C)}`

where
`C=torque\ constant`

`I=moment\ of\ inertia`

C is torque required to produce unit deflection

`C=(T)/(\theta )=(0.41)/(0.76)=0.539 N.m/rad`

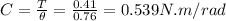
Moment of Inertia of solid sphere
`I=(2)/(5)* mr^2`

`I=(2)/(5)* 71* (0.12)^2=0.4089\ kg-m^2`

`T=2\pi \sqrt{(0.4089)/(0.539)}`

`T=2* 3.142* 0.7589`

`T=5.47 s`