Question

A 4.80 kg object is released from rest while fully submerged in a liquid. The liquid displaced by the submerged object has a mass of 2.50 kg. How far and in what direction does the object move in 0.200 s, assuming that it moves freely and that the drag force on it from the liquid is negligible?

Answer 1

According to the considerations shown at the end of the exercise, to solve this problem it is necessary to apply the kinematic equations of movement description and Newton's second law, therefore,

The force because of the weight is given by the mass and gravity, so

`F_w = mg`

`F_w = (4.8)(9.8)`

`F_w =47.04N`

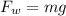
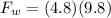
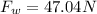
The Force due to the displaced liquid is defined as

`F_(l) = mg`

`F_(l) = (2.5)(9.8)`

`F_(l) = 24.5N`

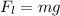
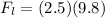
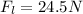
In this way the net force in the movement of the body down would be given by

`F_(net) = F_w-F_l`

`F_(net) = 47.04-24.05`

`F_(net) = 22.54N`

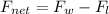
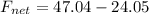
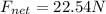
In this way the net acceleration would be,

`a = (F_(net))/(m)`

`a = (22.54)/(4.8)`

`a = 4.695m/s^2`

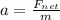
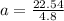
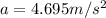
For the kinematic equations of movement description,

`x = v_i t+(1)/(2)a t^2`

`x = 0(2)+(1)/(2)(4.695)(0.2)^2`

`x = 0.0939m`

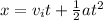
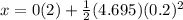
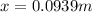
Therefore the distance traveled is 9.39cm